Fourier's Law of Heat Conduction

The Fourier's law got its name by famous mathematician and physicist Joseph Fourier. The rule is also known as the law of heat conduction.

Considering some assumption that we will see next, we can write mathematical equations as follows,

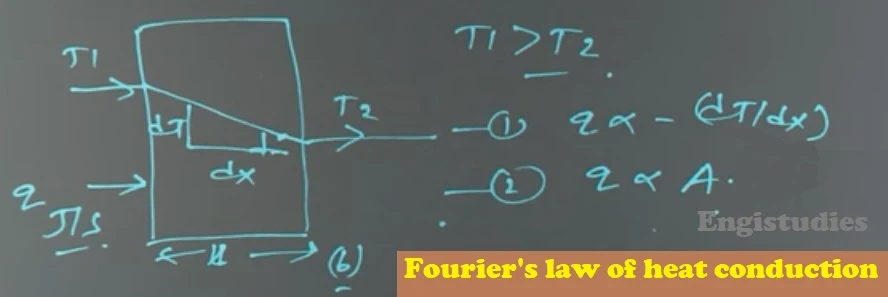
q = -k∆T = -kdT / dx

• Note that all values are in SI units.
• In the above formula, local heat flux density q is a vector obtained by the product of material conductivity k and negative local temperature gradient ∆T.

Differential form of the rule,

Q = -kA (dT / dx)

Where,

• Q = Heat rate measured in watts.
• A = The cross-sectional area that is normal to the direction of heat flow (measured in square meters)
• dT / dx = The temperature gradient (K·m⁻¹)

Note that,

• The SI unit of 'k' is W/mK (watts/meter*kelvin). To indicate the direction of 'k' vector, the negative sign is used in the formula.

The Fourier's law of thermal conduction states that,

"The time rate of heat transfer through a material is proportional to the negative gradient in the temperature and to the area."

This statement is true for all states of matter, i.e. solids, liquids, and gases.

Assumptions of Fourier's law of heat conduction

Though the law of thermal conduction is empirical, it cannot be proven mathematically, J. Fourier formulated a simple rule by analyzing the results of a number of experiments done by him in 1822.

The Fourier equation assumes the following,

• The heat flow is unidirectional and steady.
• The two faces are isothermal in nature. You can find constant and uniform temperature on both sides.
• Energy is not produced internally.
• Constant temperature gradient and linear temperature profile.